Using the CRA math strategy is an effective way to help all your students: those who are struggling with math, and those who are not.

For many students who struggle with math, it is purely psychological: fear of math or lack of math confidence. For others, it is fundamental. Maybe your students have not yet developed a strong number sense.
How can you help your students overcome these obstacles?
One great way to start is by teaching using CRA math instruction as early as possible. Pre-K students are not too young for this method. Fifth-grade students are not too old.
ALL students can benefit from learning with the CRA math strategy! Click here to get your Ultimate Guide to the CRA Math Strategy delivered right to your inbox!
What is CRA Math Strategy?
CRA stands for concrete, representative, and abstract.
- Concrete: When teaching using this framework, you start with concrete. This is an exploratory stage where students use manipulatives to construct their own background knowledge.
- Example: Students use base ten blocks to make the number 25.
- Representative: This is the second stage. Students draw or comprehend pictures that represent the concrete stage.
- Example: Students are able to draw a picture that represents the base ten blocks that are the same as 25.
- Abstract: This is the third and final stage of CRA math instruction. Students are able to explain their drawings from the representative stage using numerals and algorithms.
- Example: Students are able to correctly write the number 25.
The CRA method is used to teach most concepts in math from counting to adding, subtracting, multiplying, and dividing. It can also be used to teach fractions, decimals, and many other math concepts, as well.
Why Use CRA Math Instruction?
Using the CRA method to teach math is founded in learning theory. It is based on constructivism, introduced by psychologist Jean Piaget.
Constructivism requires students to be actively engaged in their own learning process, instead of passively absorbing information.

Benjamin Franklin once said, “Tell me and I forget. Teach me and I may remember. Involve me and I learn.” This applies to constructivism because students are involved in building their knowledge and thus develop a greater understanding of a concept.
Example: Many students can be successful if you show them a picture of 10 objects and have them count out each object. However, that can all be done through rote memorization.
Two comes after one, three comes after two, and so on and so forth.
Some students may not actually understand that two is a quantity greater than one or that four is a quantity greater than 3.
On the other hand, using the constructivist CRA math strategy, students first build out the number 25 using manipulatives like base ten blocks. Then, they draw a representation of what they built. Finally, they are able to identify the numerals that go with the number 25.
The constructivist approach is the better learning experience due to the fact that students must think critically about the number to truly understand what it means, instead of counting by rote memorization.
This helps build number sense and math confidence from a young age. However, it shouldn’t stop as students get older and math becomes more challenging.
The CRA method is used for teaching more than just counting numbers. In fact, it is also used to teach addition, subtraction, multiplication, and division, as well as fractions and decimals.
CRA Math Examples for Preschool, Early Elementary, and Upper Elementary
Below, you will find examples using the CRA math strategy for preschool, early elementary, and upper elementary concepts.
Please note that these are examples of what CRA strategy looks like and flows. It is not always appropriate to have students move through all three steps in one lesson or even one week.
Preschool: Counting to 3

- Concrete: Count out 3 objects.
- Representational: Draw 3 objects to represent the objects.
- Abstract: Write the number 3.
Early Elementary: Place Value

- Concrete: Build the number 45 using base 10 blocks using tens and ones.
- Representational: Draw a picture, showing the tens and ones.
- Abstract: Write the number 45.
Upper Elementary: Equivalent Fractions with Pattern Blocks
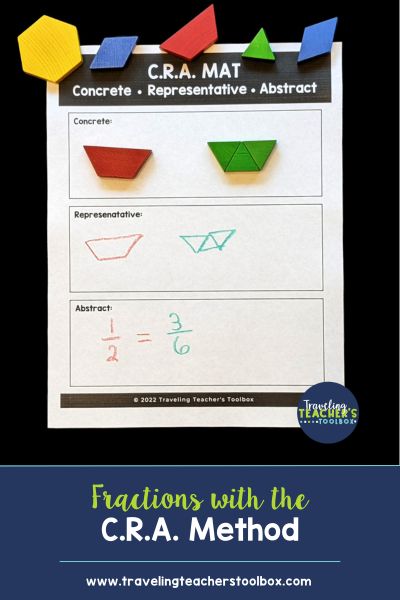
- Concrete: Using pattern blocks, show 2 different ways to build 1/2. For example, use the red block that represents 1/2 along with 3 of the green triangles that each represent 1/6.
- Representational: Draw a picture of the two different ways you built the fractions.
- Abstract: Write a number sentence showing that the two fractions are equivalent. In this case, 1/2=3/6.
Manipulatives
Many teachers shy away from the CRA method because they think you need to buy expensive materials. That is not the case! With a little creativity, you might find you already have the materials you need.
One example is that you can use rocks or pebbles as counters. Something else you could do is make paper pattern blocks by using shapes on a word processing program.
For lists of math manipulatives, click here.
Don’t have a class set? Use small groups or centers. That will reduce the quantity you need by a lot.
Unfortunately, teachers avoid using the CRA math strategy because they fear the management of materials. However, setting up and practicing procedures will help using manipulatives during math run smoothly. Likewise, it will also help your students become independent.
For more information about math manipulatives misconceptions and using manipulatives in your classroom, click here.
Final Thoughts
Using the CRA method to teach math has many benefits for all your students. It helps make math accessible for those who are struggling and helps those who are confident develop a deeper understanding.
The use of manipulatives helps students become more independent and is great for practicing fine motor skills for younger students.
The CRA method is engaging, and while it may not make all your students love math, it can help make math less scary.
Thanks for stopping by Traveling Teacher’s Toolbox!
